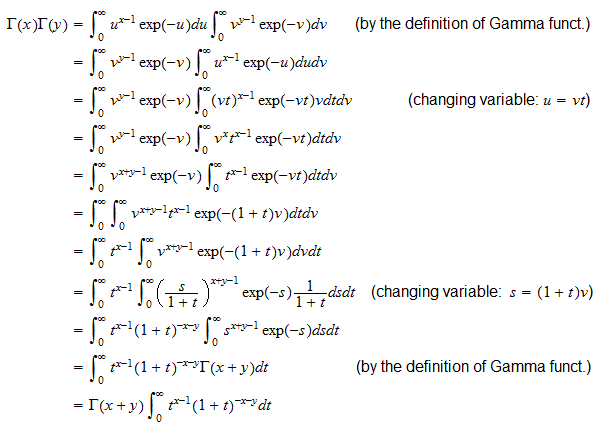
Proof Of Beta Function. It is also categorized as the Euler int. The probability density function pdf of the beta distribution for 0 x 1 and shape parameters α β 0 is a power function of the variable x and of its reflection 1 x as follows. Where Γz is the gamma functionThe beta function is a normalization constant to ensure that the total probability is 1. This article will show a particular proof for beta function by using Laplace transform and convolution formula rather than probability theory.
The distribution function F is sometimes known as the regularized incomplete beta function. An excerpt from Wikipedia How can we prove B αβ Γ α Γ β Γ αβ. Many complex integrals can be reduced to. The Beta function is the ratio of the product of the Gamma function of each parameter divided by the Gamma function of the sum of the parameters. This article will show a particular proof for beta function by using Laplace transform and convolution formula rather than probability theory. By definition the Beta function is B α β 0 1 x α 1 1 x β 1 d x where α β have real parts 0 but in this case were talking about real α β 0.
It is also categorized as the Euler int.
β 0 By evaluating 0 0 x α 1 e x y β 1 e y d x d y in two different ways show that. Just as the gamma function for integers describes fac-torials the beta function can dene a. Beta Function and its Applications Riddhi D. Where Bab B a b is the beta function and Bxab B x. It is also categorized as the Euler int. The beta function Buv is also de ned by means of an integral.